Duration analysis measures the change in the valuation of an asset or liability that may occur given a discrete change in interest rates. It is a very useful concept for understanding how the value of an instrument, portfolio, or even balance sheet will change for a specified percentage move in market rates. For example, if duration is calculated to be 3.8, this means for a 1 percent increase in interest rates, a bond’s price is expected to decline by 3.8 percent. The analysis will factor in the size and timing of cash flows occurring before the contractual maturity of an asset or liability. Duration is the price volatility of a zero-coupon bond with that number of years to maturity. For example, if a bond has a duration of four years, it has the same approximate price sensitivity to rate changes as a four-year zero-coupon bond.
Duration underpins contemporary portfolio and balance sheet management theories. While it may not be used as a direct policy limit parameter, it can be a useful measurement to quickly understand a portfolio’s sensitivity to changes in interest rates. Beyond its utility as an instrument level measurement, duration can be aggregated on a weighted basis across portfolios or the entire balance sheet and provides important insight into overall price sensitivity. For example, measurements such as NEV provide a capital-at-risk approach that is rooted in the duration mismatches of the underlying balance sheet portfolios. In some cases, the economic value of balance sheet equity can also be expressed in terms of duration (duration of equity).
Duration of equity is derived from subtracting the duration of total liabilities from the duration of total assets. The longer the duration of equity, the more sensitive it becomes to a given change in rates. In this way, duration can be used as a proxy for overall price and value sensitivity.
There are three basic determinants that will affect a financial instrument’s duration:
- Maturity – the longer the maturity of the bond, the higher the duration
- Cash flows – the more front-loaded the cash flows, the lower the duration
- Coupon – the higher the coupon of the instrument, the lower the duration
Duration allows instruments of different maturities and coupon rates to be directly compared. The higher the duration, the higher the risk of price changes as interest rates change. A credit union can build its portfolio based on weighted average duration, which provides the ability to determine portfolio value changes based on a forecasted change in interest rates.
There are three customary measures of duration: Macaulay, modified, and effective.
Macaulay Duration calculates the weighted average term to maturity of an investment’s cash flows. Duration, stated in months or years, will:
-
Equal less than maturity for investments with payments before maturity
-
Decline as time passes
-
Equal maturity for zero-coupon investments
-
Really makes sense only for an instrument with fixed cash flows
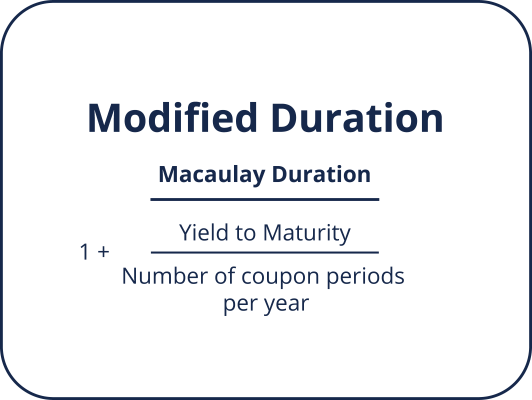
Modified Duration will use the calculation from the Macaulay duration, and estimate price sensitivity for small interest rate changes. An investment’s modified duration represents its percentage price change given a small change in interest rates.
Modified duration assumes that interest rate shifts will not change an investment’s cash flows. As a result, it does not estimate price sensitivity with an acceptable level of precision for option-based assets.
Effective Duration estimates price sensitivity more accurately than modified duration for instruments with embedded options, and is calculated using valuation models that contain option-pricing components. First, a credit union must determine the financial instrument’s current value. The valuation model then assumes an interest rate change (usually 100 basis points) and estimates the instrument’s new value based on that assumption. The percentage change between the current and forecasted values represents the instrument’s effective duration.
All duration measures will assume a linear price/yield relationship; however, larger shifts in rates will have a greater effect than smaller shifts. Therefore, duration may only accurately estimate price sensitivity for rather small (up to 100 basis point) interest rate changes.
Last updated October 11, 2016